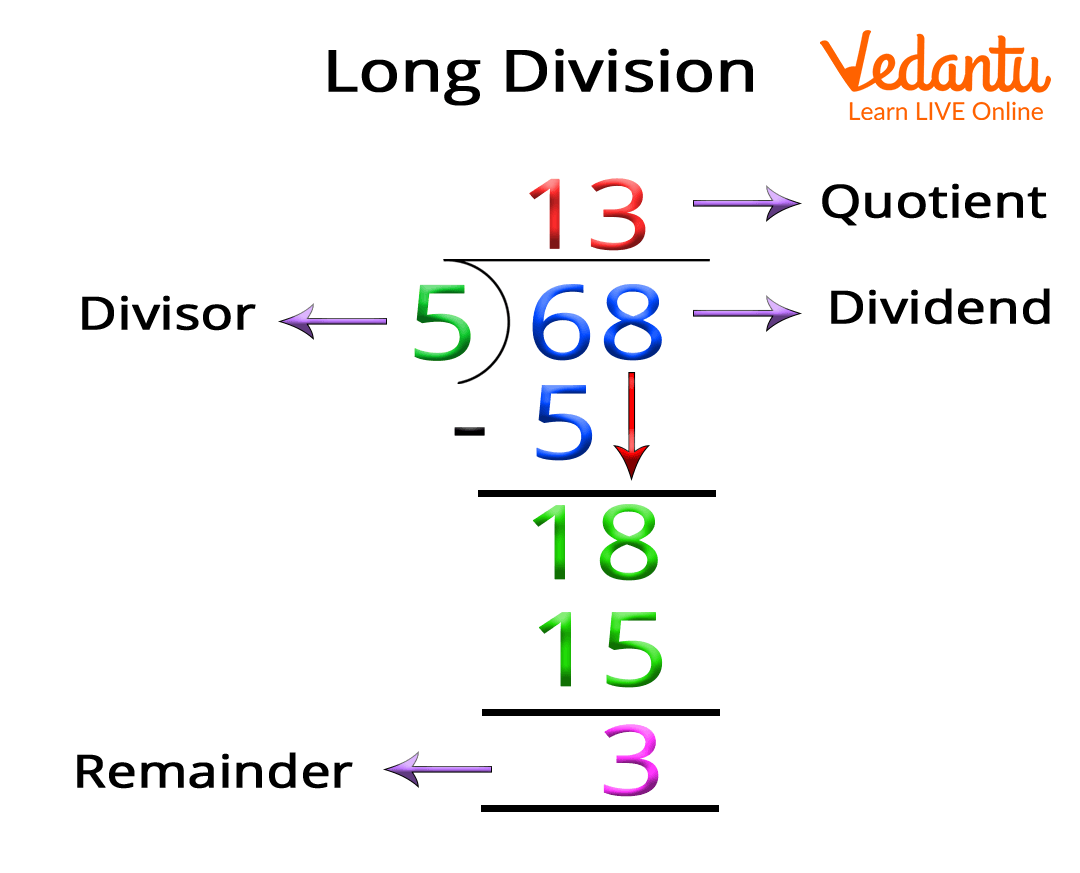
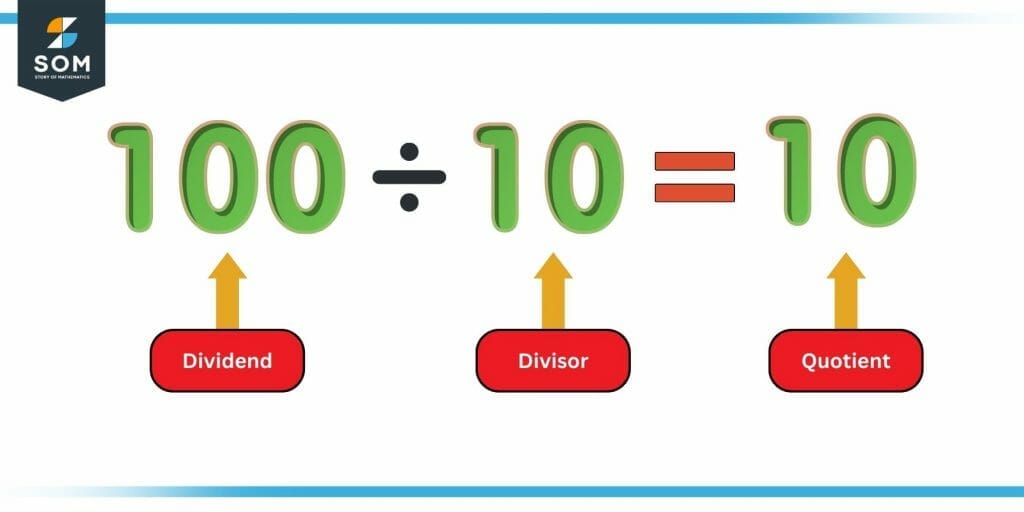
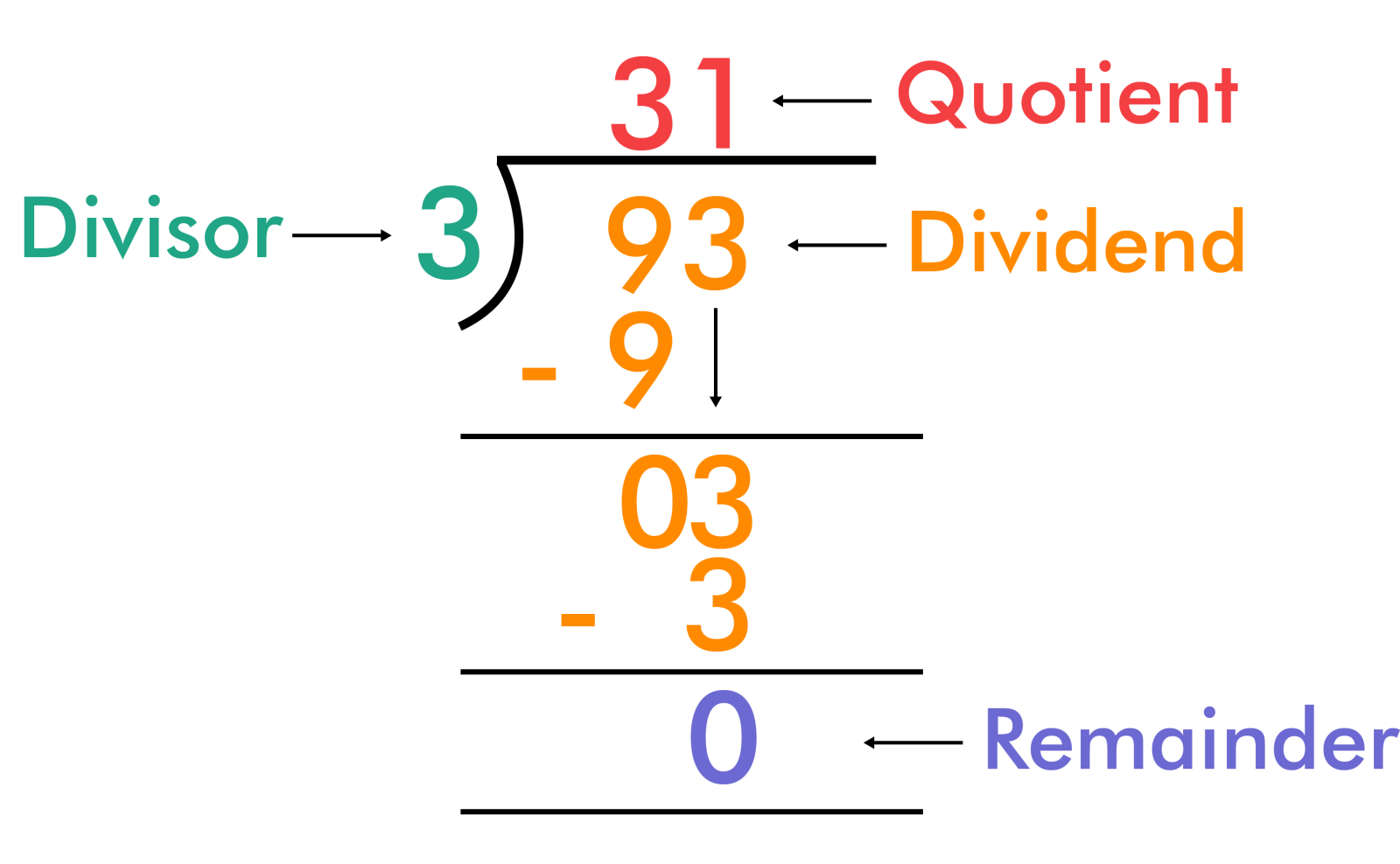Quotient Remainder Form - When we divide 13 ÷ 4, the remainder is. Given any integer n and a positive integer d, there exist unique integers q and r such that: When dividends are not split evenly by the divisor, then the leftover part is the remainder. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b : N = d⋅q + r, and 0 ≤ r <.
When dividends are not split evenly by the divisor, then the leftover part is the remainder. Given any integer n and a positive integer d, there exist unique integers q and r such that: When we divide 13 ÷ 4, the remainder is. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b : N = d⋅q + r, and 0 ≤ r <.
Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b : When dividends are not split evenly by the divisor, then the leftover part is the remainder. N = d⋅q + r, and 0 ≤ r <. Given any integer n and a positive integer d, there exist unique integers q and r such that: When we divide 13 ÷ 4, the remainder is.
Division With Remainders Examples
N = d⋅q + r, and 0 ≤ r <. When dividends are not split evenly by the divisor, then the leftover part is the remainder. Given any integer n and a positive integer d, there exist unique integers q and r such that: When we divide 13 ÷ 4, the remainder is. Quotient function given two integers a ,.
What is a Remainder in Math? (Definition, Examples) BYJUS
Given any integer n and a positive integer d, there exist unique integers q and r such that: N = d⋅q + r, and 0 ≤ r <. When we divide 13 ÷ 4, the remainder is. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r.
Remainder Definition, Facts & Examples
Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b : Given any integer n and a positive integer d, there.
Question Video Finding a Quotient and Remainder from a Polynomial
When we divide 13 ÷ 4, the remainder is. N = d⋅q + r, and 0 ≤ r <. When dividends are not split evenly by the divisor, then the leftover part is the remainder. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ.
Writing Polynomials in (Divisor)(Quotient) + Remainder Form
When dividends are not split evenly by the divisor, then the leftover part is the remainder. When we divide 13 ÷ 4, the remainder is. N = d⋅q + r, and 0 ≤ r <. Given any integer n and a positive integer d, there exist unique integers q and r such that: Quotient function given two integers a ,.
PPT Division of Polynomials PowerPoint Presentation ID272069
When dividends are not split evenly by the divisor, then the leftover part is the remainder. N = d⋅q + r, and 0 ≤ r <. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such.
What is a Remainder in Math? (Definition, Examples) BYJUS
N = d⋅q + r, and 0 ≤ r <. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b :.
Quotient Definition & Meaning
When we divide 13 ÷ 4, the remainder is. N = d⋅q + r, and 0 ≤ r <. Given any integer n and a positive integer d, there exist unique integers q and r such that: When dividends are not split evenly by the divisor, then the leftover part is the remainder. Quotient function given two integers a ,.
The Remainder Theorem Top Online General
Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b : When dividends are not split evenly by the divisor, then.
How to write it in quotient + remainder/divisor form
When dividends are not split evenly by the divisor, then the leftover part is the remainder. N = d⋅q + r, and 0 ≤ r <. Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such.
N = D⋅Q + R, And 0 ≤ R <.
When dividends are not split evenly by the divisor, then the leftover part is the remainder. Given any integer n and a positive integer d, there exist unique integers q and r such that: Quotient function given two integers a , b ∈ ℤ such that b ≠ 0 , then we get some q , r ∈ ℤ with 0 ≤ r < | b | such that a = b · q + r and we define a / / b : When we divide 13 ÷ 4, the remainder is.