Phase Variable Form - If m < n (strictly proper), then bn = 0, ci = bi. In this form, the coefficients of the characteristic polynomial appear in the last row. This structure is known as phase variable canonical form (pvcf). The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. The proof follows immediately upon carrying out the indicated change of. It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),.
In this form, the coefficients of the characteristic polynomial appear in the last row. The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. If m < n (strictly proper), then bn = 0, ci = bi. It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. The proof follows immediately upon carrying out the indicated change of. This structure is known as phase variable canonical form (pvcf).
If m < n (strictly proper), then bn = 0, ci = bi. This structure is known as phase variable canonical form (pvcf). The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. The proof follows immediately upon carrying out the indicated change of. In this form, the coefficients of the characteristic polynomial appear in the last row. It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),.
Controllable Canonical Phase Variable Form Method 1 Converting
This structure is known as phase variable canonical form (pvcf). In this form, the coefficients of the characteristic polynomial appear in the last row. It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. The proof follows immediately upon.
Feedback Control Systems (FCS) ppt download
It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. If m < n (strictly proper), then bn = 0, ci.
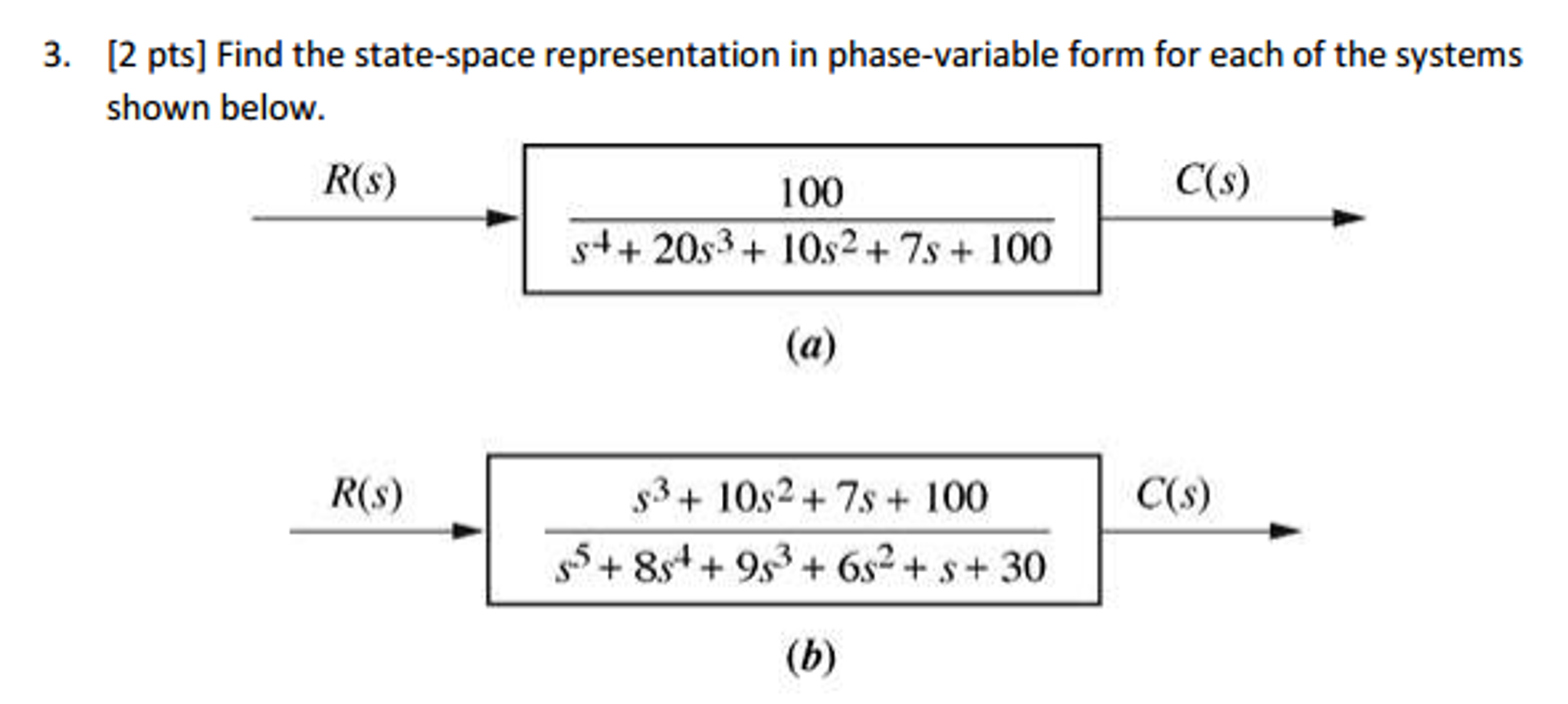
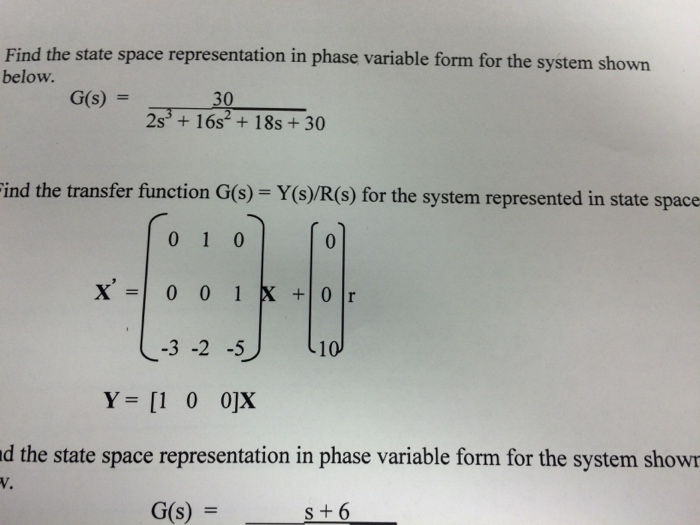
Solved Find the statespace representation in phasevariable
In this form, the coefficients of the characteristic polynomial appear in the last row. This structure is known as phase variable canonical form (pvcf). It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. The proof follows immediately upon.
PPT Feedback Control Systems (FCS) PowerPoint Presentation, free
If m < n (strictly proper), then bn = 0, ci = bi. The proof follows immediately upon carrying out the indicated change of. In this form, the coefficients of the characteristic polynomial appear in the last row. The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. This structure is known.
State Space Representation in Phase Variable Form Lec2 YouTube
This structure is known as phase variable canonical form (pvcf). In this form, the coefficients of the characteristic polynomial appear in the last row. The proof follows immediately upon carrying out the indicated change of. If m < n (strictly proper), then bn = 0, ci = bi. It is common to express the state equations in a vector form,.
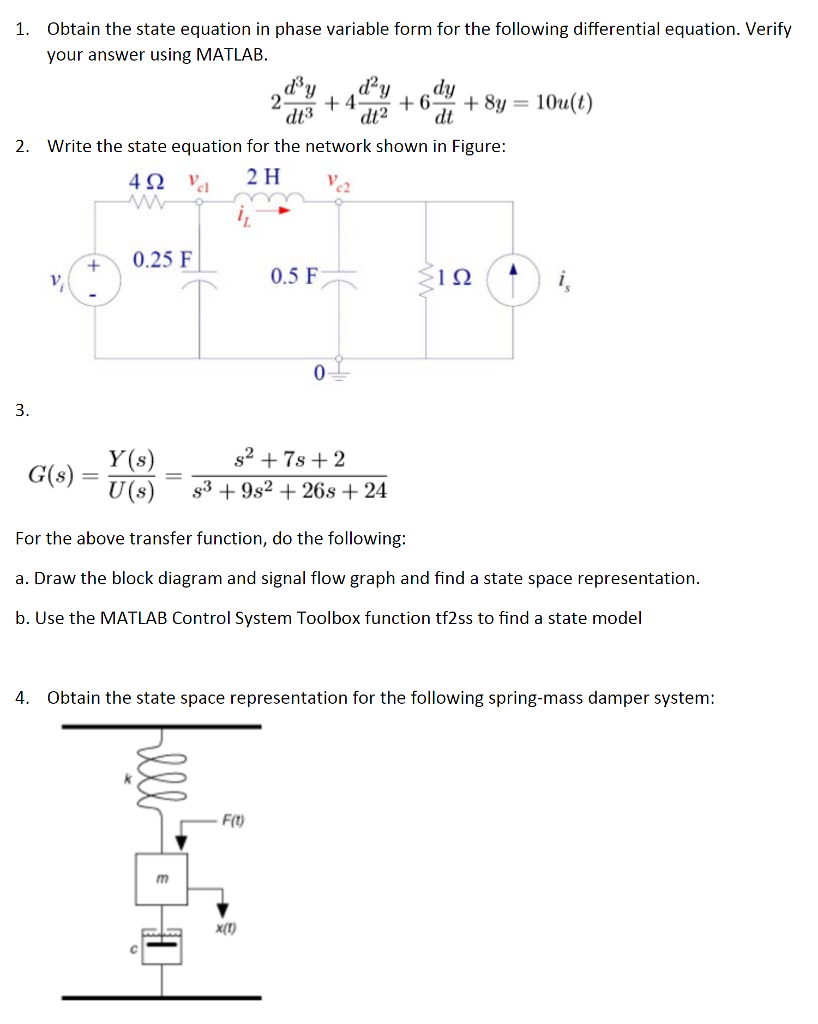
Solved 1. Obtain the state equation in phase variable form
The proof follows immediately upon carrying out the indicated change of. If m < n (strictly proper), then bn = 0, ci = bi. This structure is known as phase variable canonical form (pvcf). It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector.
Phase Variable form from State Space Myacademy YouTube
It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. If m < n (strictly proper), then bn = 0, ci = bi. The phase variable form is obtained simply by renumbering the phase variables in the opposite order.
Solved Find The State Space Representation In Phase Varia...
If m < n (strictly proper), then bn = 0, ci = bi. This structure is known as phase variable canonical form (pvcf). The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. The proof follows immediately upon carrying out the indicated change of. In this form, the coefficients of the characteristic.
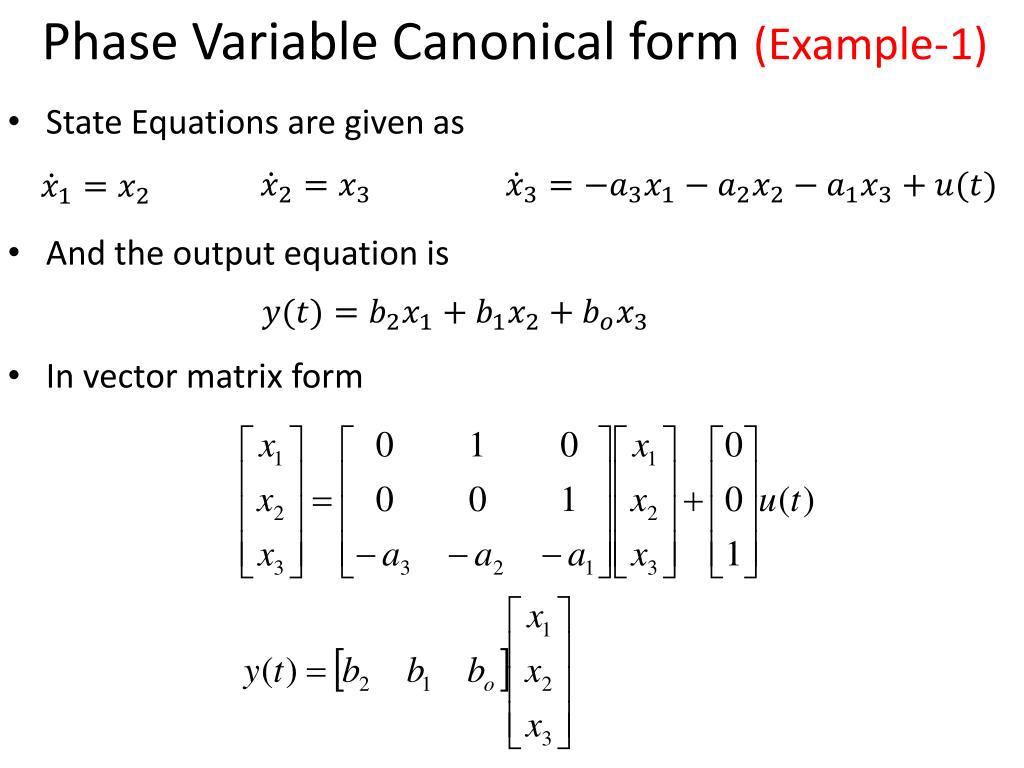
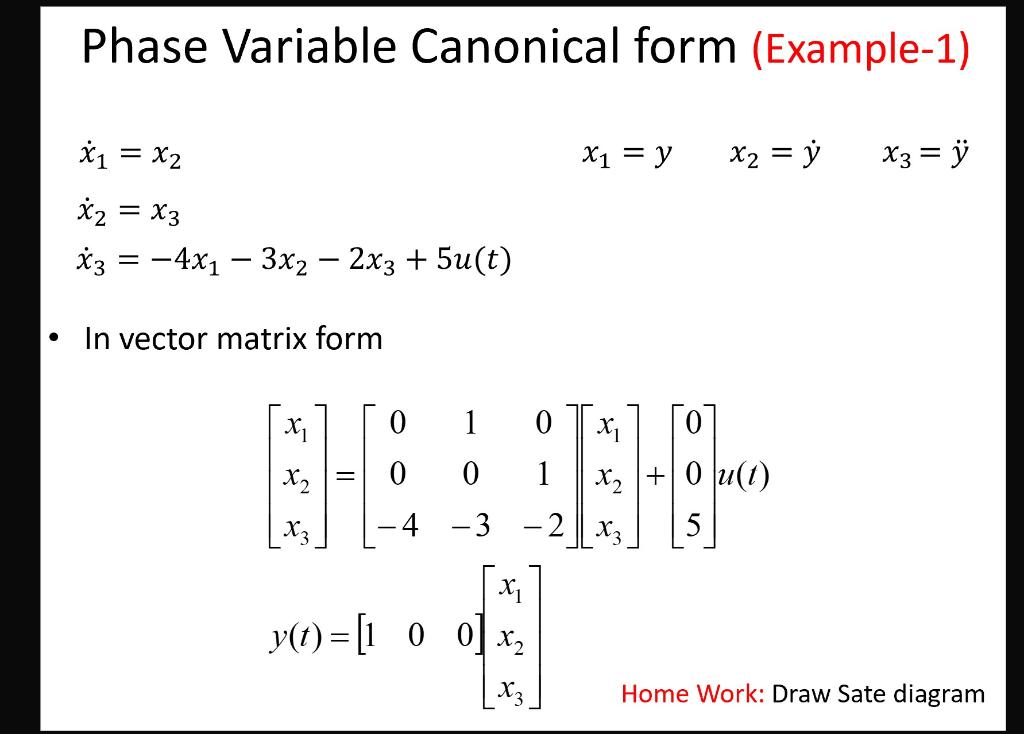
Solved Phase Variable Canonical form (Example1)
This structure is known as phase variable canonical form (pvcf). The proof follows immediately upon carrying out the indicated change of. If m < n (strictly proper), then bn = 0, ci = bi. The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. It is common to express the state equations.
Lecture 3 State Space Canonical forms YouTube
If m < n (strictly proper), then bn = 0, ci = bi. In this form, the coefficients of the characteristic polynomial appear in the last row. It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. This structure.
This Structure Is Known As Phase Variable Canonical Form (Pvcf).
It is common to express the state equations in a vector form, in which the set of n state variables is written as a state vector x(t) = [x1(t), x 2(t),. In this form, the coefficients of the characteristic polynomial appear in the last row. The phase variable form is obtained simply by renumbering the phase variables in the opposite order of the. If m < n (strictly proper), then bn = 0, ci = bi.