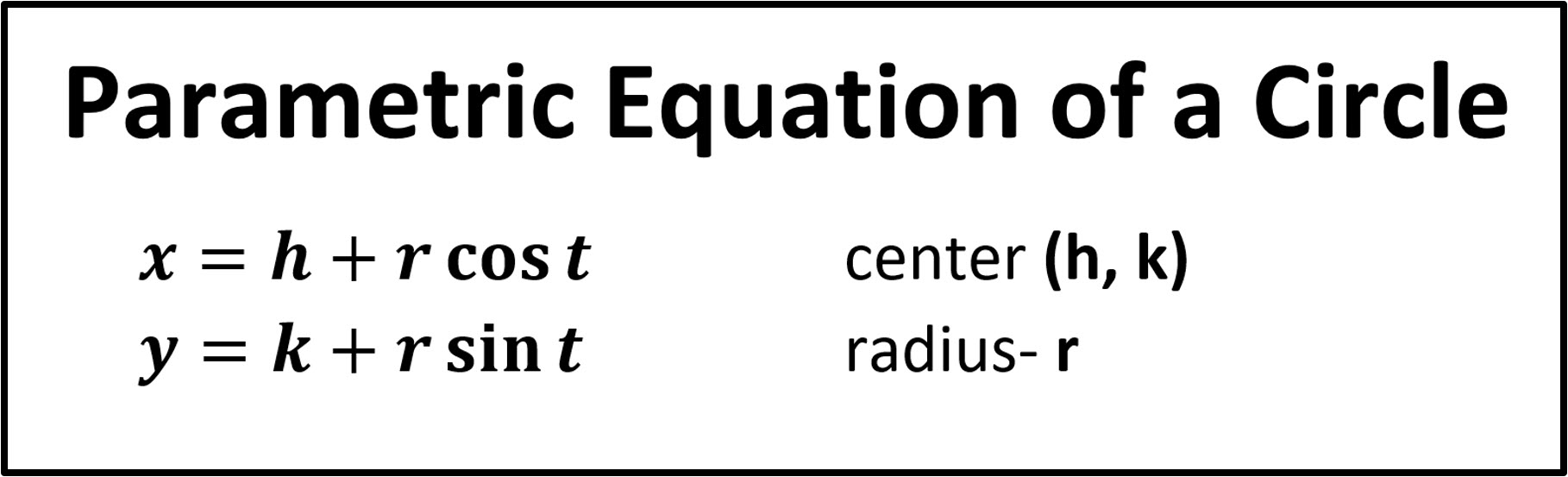
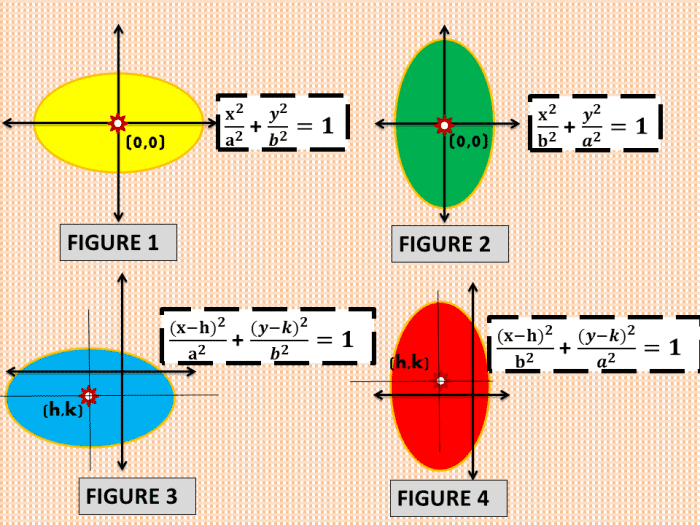
Parametric Form Of An Ellipse - The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? This is done by expanding the sines and forming. I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as:
Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; This is done by expanding the sines and forming. I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as:
Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: This is done by expanding the sines and forming. The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients;
Ex Find Parametric Equations For Ellipse Using Sine And Cosine From a
The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so.
Normal of an Ellipse L9 Three Equations 1 Parametric form 2 Point
The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; This is done by expanding the sines and forming. I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: Consider the ellipse given by \(\frac{x^2}{9}.
Equation of Ellipse in parametric form
I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; This is done by expanding the sines and forming. Consider the ellipse given by \(\frac{x^2}{9}.
Wie man eine Ellipse mit einer gegebenen Gleichung grafisch darstellt
I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: This is done by expanding the sines and forming. The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; Consider the ellipse given by \(\frac{x^2}{9}.
S 2.26 Parametric Equation of Ellipse How to Find Parametric Equation
Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: This is done by expanding the sines and forming. The general form of this ellipse is $$a x^2 + b x y.
Ellipse Equation, Properties, Examples Ellipse Formula
This is done by expanding the sines and forming. Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: The general form of this ellipse is $$a x^2 + b x y.
How to Write the Parametric Equations of an Ellipse in Rectangular Form
This is done by expanding the sines and forming. The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: Consider the ellipse given by \(\frac{x^2}{9}.
Parametric Equations Conic Sections
I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: This is done by expanding the sines and forming. Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? The general form of this ellipse is $$a x^2 + b x y.
How to Graph an Ellipse Given an Equation Owlcation
I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? This is done by expanding the sines and forming. The general form of this ellipse is $$a x^2 + b x y.
tangent at vertex of ellipse, parametric form, focal length, auxiliary ci..
This is done by expanding the sines and forming. I know that $a=2$ and $b=1$ (where $a$ and $b$ are the axis of the ellipse), so i parameterize as: Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? The general form of this ellipse is $$a x^2 + b x y.
I Know That $A=2$ And $B=1$ (Where $A$ And $B$ Are The Axis Of The Ellipse), So I Parameterize As:
The general form of this ellipse is $$a x^2 + b x y + c y^2 = 1$$ the idea is to find the coefficients; Consider the ellipse given by \(\frac{x^2}{9} + \frac{y^2}{4} = 1.\) what are the parametric equations for this ellipse? This is done by expanding the sines and forming.