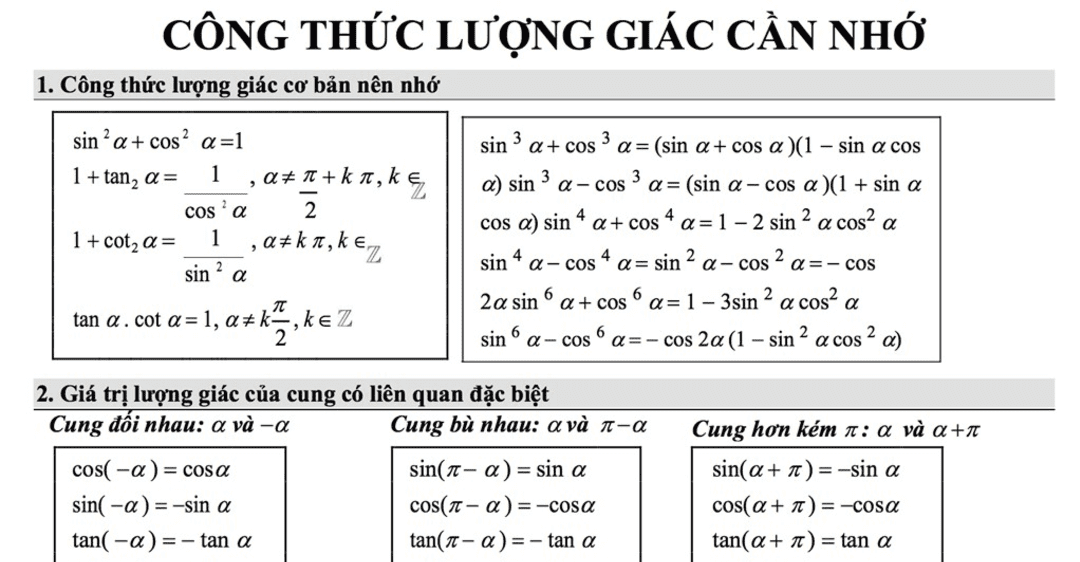
If Cos Is 1 3 What Is Sin - Given these values, we can work out the adj side of our imaginary triangle to work out cosθ. Sinθ = opp hyp, in this case 1 3. Can you find the adjacent side through pythagoras'. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. The quadrant determines the sign on each of the values. Use the definition of cosine to find the known sides of the unit circle right triangle. Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse. Sin (x) = ± 1 − 9 1 = ± 3 8.
Can you find the adjacent side through pythagoras'. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. Use the definition of cosine to find the known sides of the unit circle right triangle. Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse. Given these values, we can work out the adj side of our imaginary triangle to work out cosθ. Sin (x) = ± 1 − 9 1 = ± 3 8. The quadrant determines the sign on each of the values. Sinθ = opp hyp, in this case 1 3.
Can you find the adjacent side through pythagoras'. Use the definition of cosine to find the known sides of the unit circle right triangle. Sin (x) = ± 1 − 9 1 = ± 3 8. Given these values, we can work out the adj side of our imaginary triangle to work out cosθ. The quadrant determines the sign on each of the values. Sinθ = opp hyp, in this case 1 3. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse.
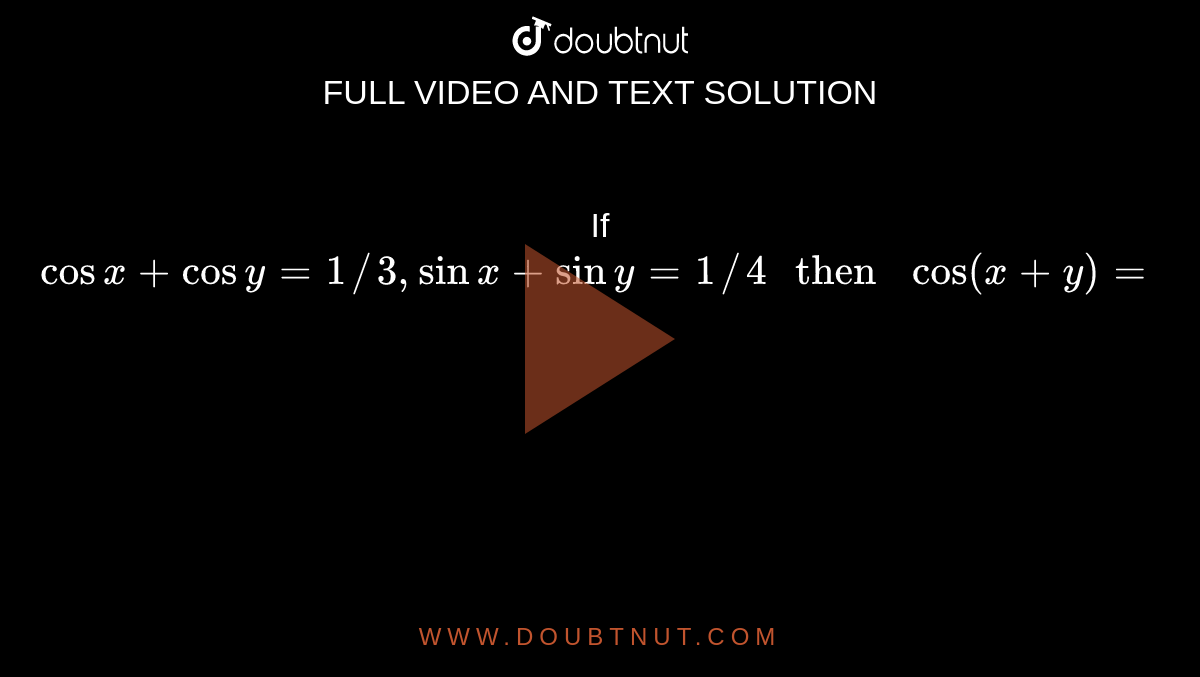
If cos x+ cos y = 1//3 , sin x + sin y=1//4 " then " cos (x+y)=
Given these values, we can work out the adj side of our imaginary triangle to work out cosθ. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. Sin (x) = ± 1 −.
sin[cos^(1)(3/5)]
Can you find the adjacent side through pythagoras'. Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has.
Cosine And Sine Graph
Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse. Can you find the adjacent side through pythagoras'. The quadrant determines the sign on each of the values. Sin (x) = ± 1 − 9 1 = ± 3 8. Given these values, we can work out the adj side of our imaginary.
Sin and Cos Graphs XavierhasTran
Given these values, we can work out the adj side of our imaginary triangle to work out cosθ. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. The quadrant determines the sign on.
sin inverse x + cos inverse (1 x)=sin inverse ( x)
The quadrant determines the sign on each of the values. Sinθ = opp hyp, in this case 1 3. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. Given these values, we can.
Question 5 If sin (sin1 1/5 + cos1 x) = 1, find x CBSE
Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse. Can you find the adjacent side through pythagoras'. The quadrant determines the sign on each of the values. Sin (x) = ± 1 − 9 1 = ± 3 8. Sinθ = opp hyp, in this case 1 3.
Công thức Sin Cos Khám phá Toàn Diện từ Cơ Bản đến Nâng Cao
Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. Sinθ = opp hyp, in this case 1 3. Let theta be an angle, 1 will be the opposite side, and 3 will be.
Example 10 Show that sin1 3/5 sin1 8/17 = cos1 84/85
Sinθ = opp hyp, in this case 1 3. The quadrant determines the sign on each of the values. Use the definition of cosine to find the known sides of the unit circle right triangle. Sin (x) = ± 1 − 9 1 = ± 3 8. Therefore, if cos ( x ) = 1 / 3 \cos(x) =.
Misc 6 Prove cos1 12/13 + sin1 3/5 = sin1 56/65 Miscellaneous
Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. The quadrant determines the sign on each of the values. Use the definition of cosine to find the known sides of the unit circle.
Solved prove that sin cot^(1) tan cos^(1) 3/4 = 3/4 [algebra]
Given these values, we can work out the adj side of our imaginary triangle to work out cosθ. Sinθ = opp hyp, in this case 1 3. Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x.
Can You Find The Adjacent Side Through Pythagoras'.
Sin (x) = ± 1 − 9 1 = ± 3 8. Use the definition of cosine to find the known sides of the unit circle right triangle. Let theta be an angle, 1 will be the opposite side, and 3 will be the hypotenuse. Given these values, we can work out the adj side of our imaginary triangle to work out cosθ.
The Quadrant Determines The Sign On Each Of The Values.
Therefore, if cos ( x ) = 1 / 3 \cos(x) = 1/3 cos ( x ) = 1/3 , we conclude that sin ( x ) \sin(x) sin ( x ) has two. Sinθ = opp hyp, in this case 1 3.
![sin[cos^(1)(3/5)]](https://static.doubtnut.com/ss/web/1130785.webp)