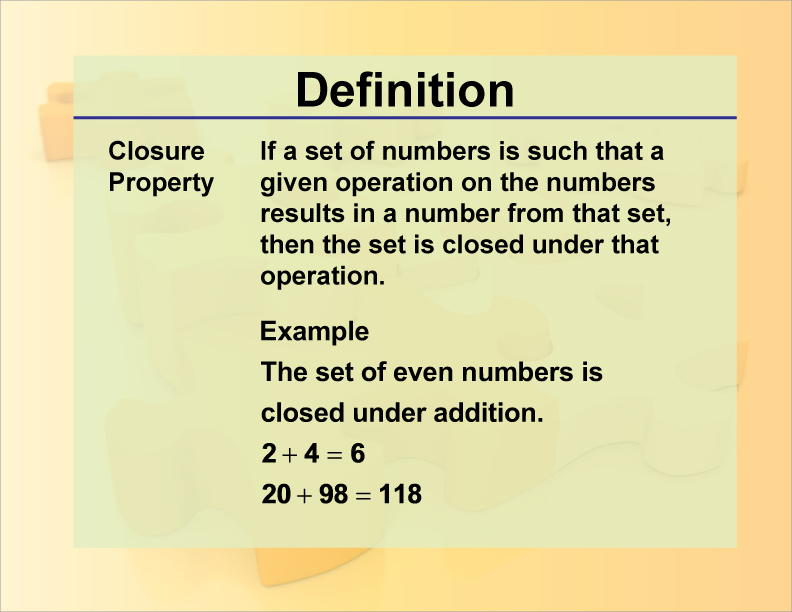
Define Closure Property In Math - In mathematics, closure refers to the likelihood of an operation on elements of a set. If something is closed, then it means if an operation is. The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. Closure property of whole numbers under addition: Closure property holds for addition and multiplication of whole numbers. What is the closure property?
Closure property of whole numbers under addition: What is the closure property? If something is closed, then it means if an operation is. Closure property holds for addition and multiplication of whole numbers. The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. In mathematics, closure refers to the likelihood of an operation on elements of a set.
Closure property of whole numbers under addition: If something is closed, then it means if an operation is. What is the closure property? In mathematics, closure refers to the likelihood of an operation on elements of a set. Closure property holds for addition and multiplication of whole numbers. The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique.
Algebra 1 2.01d The Closure Property YouTube
In mathematics, closure refers to the likelihood of an operation on elements of a set. Closure property holds for addition and multiplication of whole numbers. What is the closure property? If something is closed, then it means if an operation is. The closure property of addition for real numbers states that if a and b are real numbers, then a.
8 Math Rational numbers Closure property YouTube
The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. Closure property of whole numbers under addition: If something is closed, then it means if an operation is. Closure property holds for addition and multiplication of whole numbers. In mathematics, closure refers to the likelihood of.
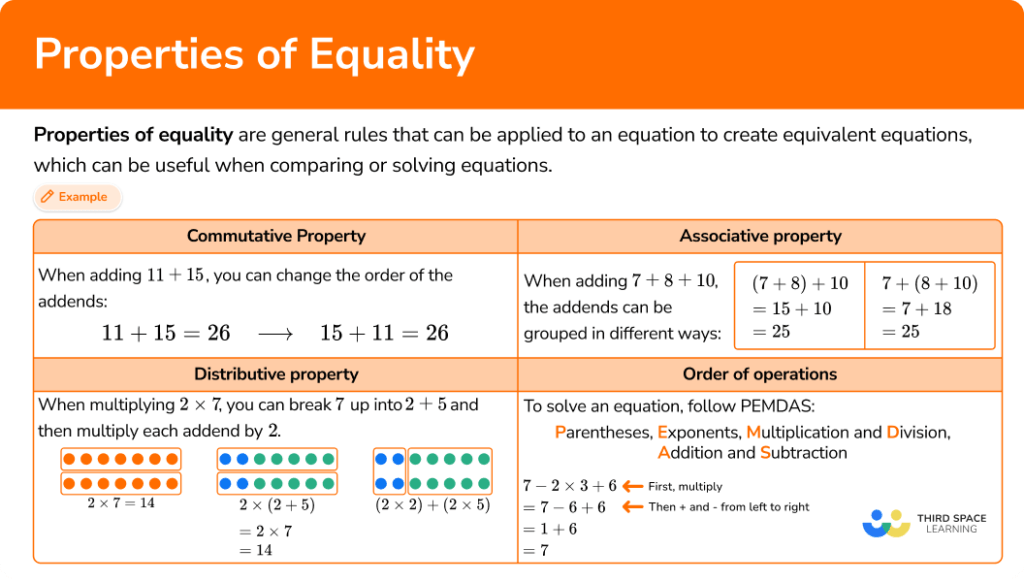
Properties of Equality Math Steps, Examples & Questions
In mathematics, closure refers to the likelihood of an operation on elements of a set. What is the closure property? Closure property of whole numbers under addition: The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. If something is closed, then it means if an.
Closure Property Of Addition Definition slidesharetrick
Closure property of whole numbers under addition: In mathematics, closure refers to the likelihood of an operation on elements of a set. If something is closed, then it means if an operation is. The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. What is the.
Properties of Integers Closure Property MathsMD
Closure property of whole numbers under addition: If something is closed, then it means if an operation is. What is the closure property? The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. In mathematics, closure refers to the likelihood of an operation on elements of.
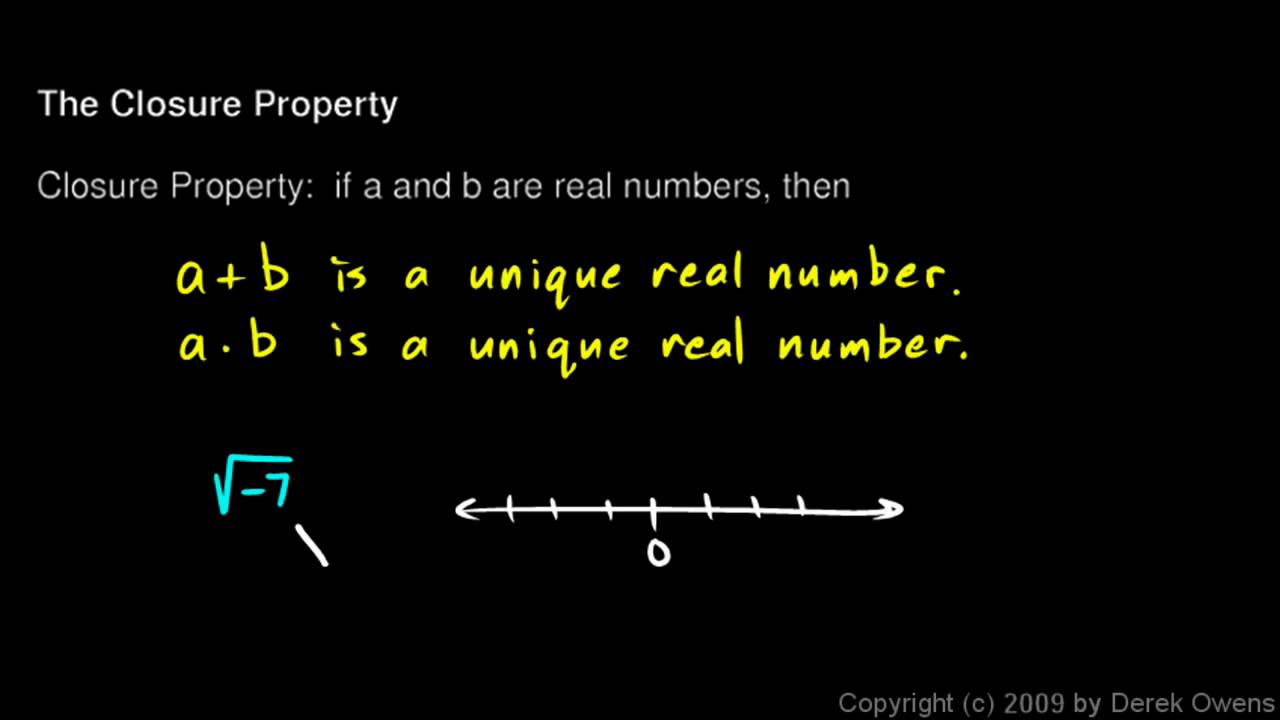
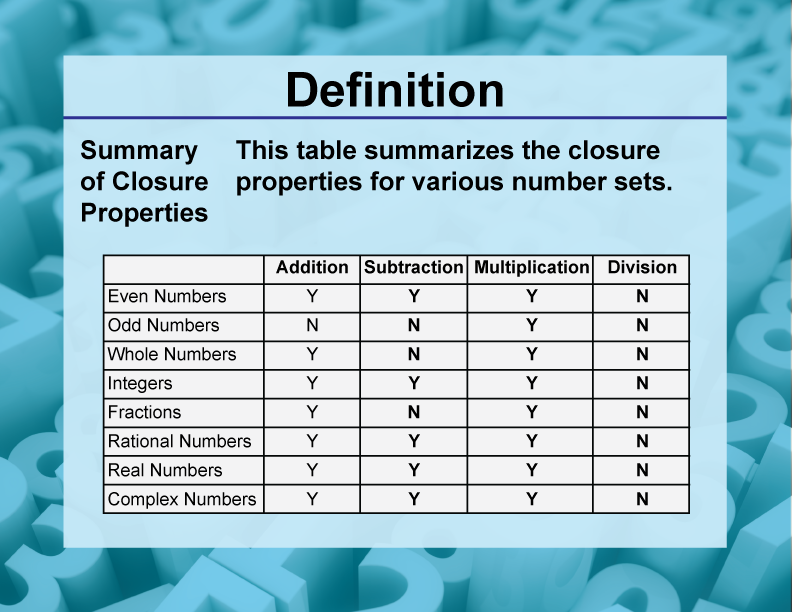
DefinitionClosure Property TopicsSummary of Closure Properties
Closure property holds for addition and multiplication of whole numbers. If something is closed, then it means if an operation is. The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. What is the closure property? Closure property of whole numbers under addition:
Closure Property Of Addition Definition slidesharetrick
Closure property of whole numbers under addition: In mathematics, closure refers to the likelihood of an operation on elements of a set. If something is closed, then it means if an operation is. What is the closure property? The closure property of addition for real numbers states that if a and b are real numbers, then a + b is.
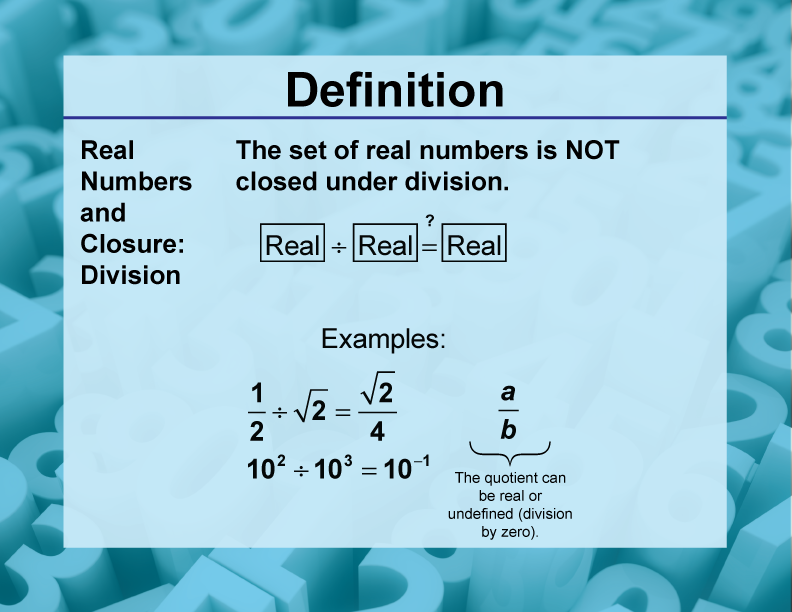
DefinitionClosure Property TopicsReal Numbers and Closure Division
If something is closed, then it means if an operation is. What is the closure property? In mathematics, closure refers to the likelihood of an operation on elements of a set. Closure property holds for addition and multiplication of whole numbers. The closure property of addition for real numbers states that if a and b are real numbers, then a.
Closure of Relations (Solved Problems) Set 2 YouTube
If something is closed, then it means if an operation is. What is the closure property? In mathematics, closure refers to the likelihood of an operation on elements of a set. Closure property of whole numbers under addition: Closure property holds for addition and multiplication of whole numbers.
DefinitionClosure Property Media4Math
The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. What is the closure property? Closure property holds for addition and multiplication of whole numbers. In mathematics, closure refers to the likelihood of an operation on elements of a set. Closure property of whole numbers under.
Closure Property Holds For Addition And Multiplication Of Whole Numbers.
What is the closure property? In mathematics, closure refers to the likelihood of an operation on elements of a set. The closure property of addition for real numbers states that if a and b are real numbers, then a + b is a unique. If something is closed, then it means if an operation is.