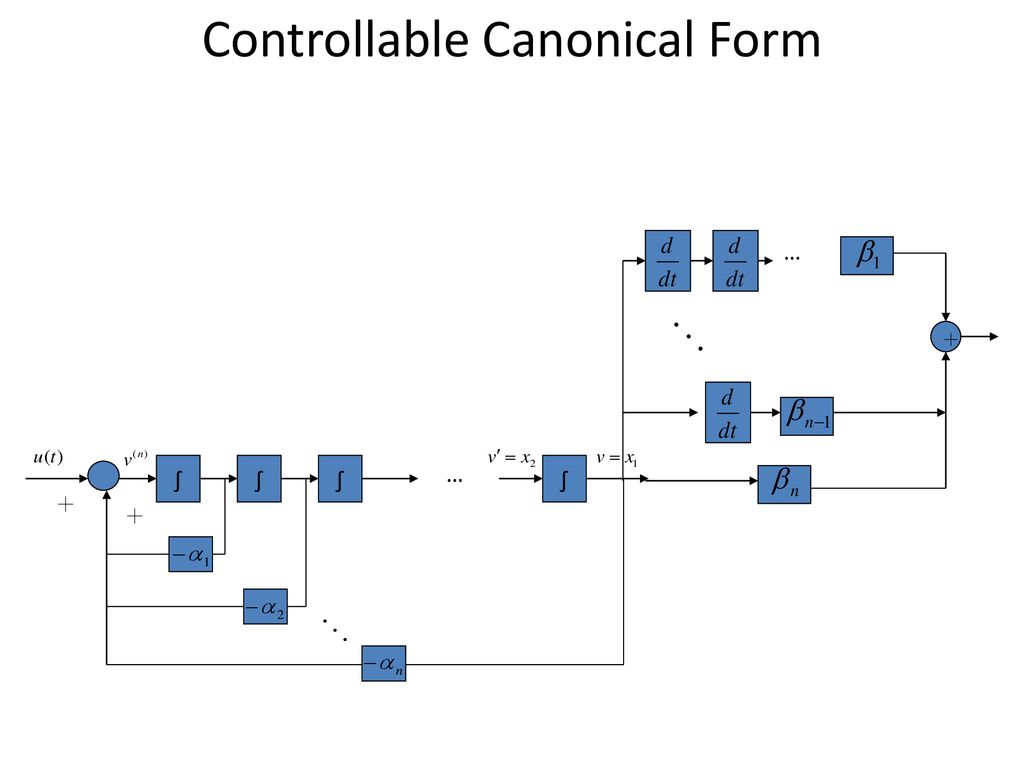
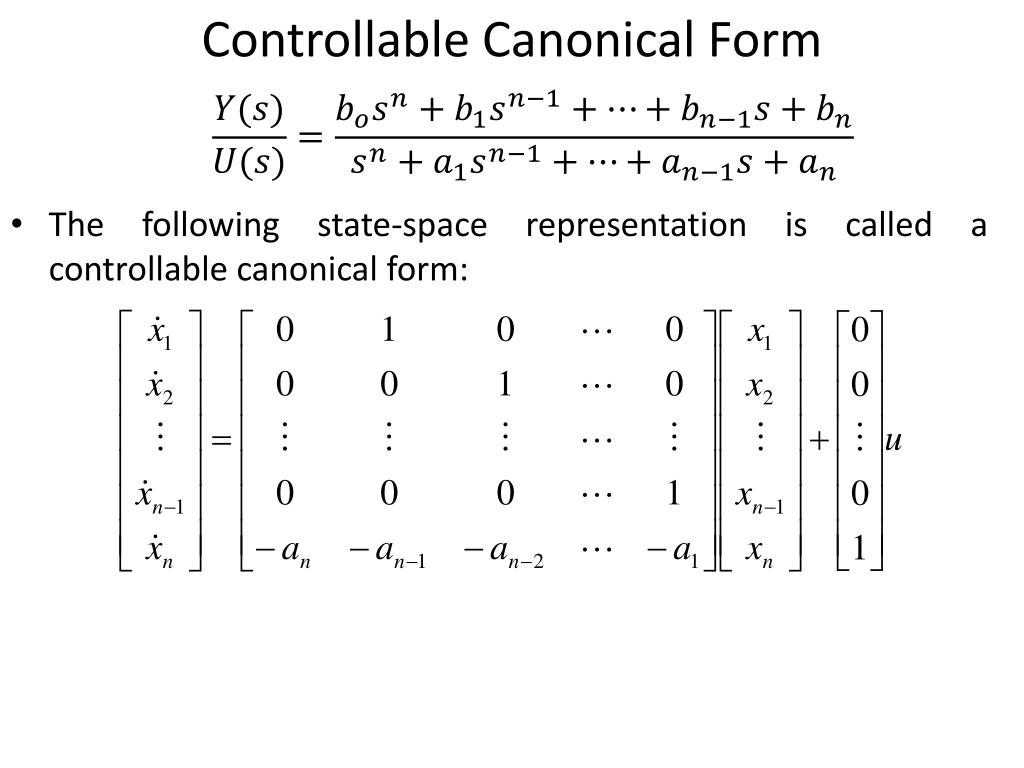
Controllable Canonical Form - We will see that there are multiple models (or realizations) that correspond to the same transfer function. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. This realization is called the controllable canonical form uw linear systems (x.
Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. This realization is called the controllable canonical form uw linear systems (x. We will see that there are multiple models (or realizations) that correspond to the same transfer function.
Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. We will see that there are multiple models (or realizations) that correspond to the same transfer function. This realization is called the controllable canonical form uw linear systems (x. Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the.
Easy Explanation of Controllable Canonical Form Control Engineering
Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. This realization is called the controllable canonical form uw linear systems (x. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using..
PPT Feedback Control Systems (FCS) PowerPoint Presentation, free
We will see that there are multiple models (or realizations) that correspond to the same transfer function. Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial.
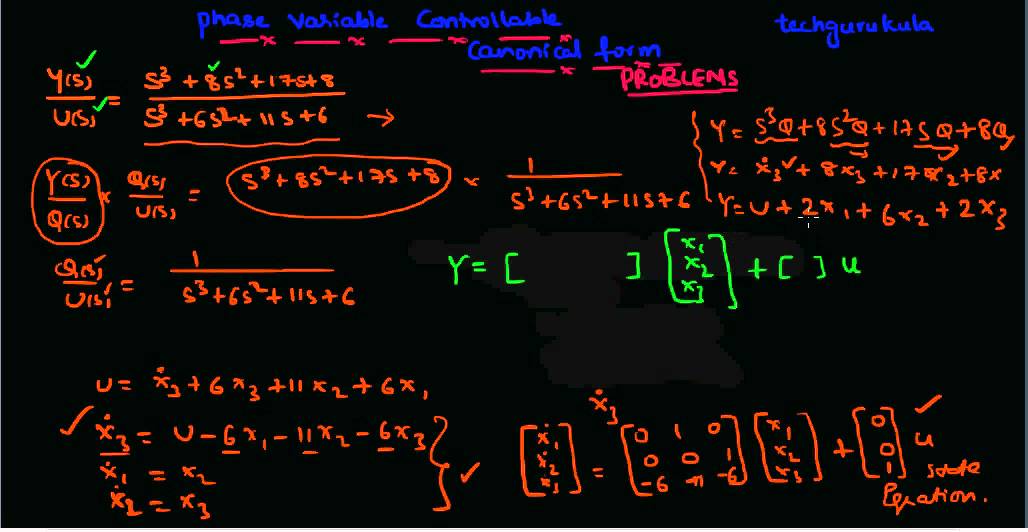
controllable canonical form problem solving YouTube
Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. This realization is called the controllable canonical form uw linear systems (x. We will see that there are multiple models (or realizations) that correspond to the same transfer function. Learn how to obtain controllable.
Feedback Control Systems (FCS) ppt download
We will see that there are multiple models (or realizations) that correspond to the same transfer function. This realization is called the controllable canonical form uw linear systems (x. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. Theorem (kalman canonical form (controllability)) let x 2rn, x(k.
Feedback Control Systems (FCS) ppt download
We will see that there are multiple models (or realizations) that correspond to the same transfer function. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. This realization is called the controllable canonical form uw linear systems (x. Theorem (kalman canonical form (controllability)) let x 2rn, x(k.
Feedback Control Systems (FCS) ppt download
Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. We will see that there are multiple models (or realizations) that correspond to the same transfer function. This realization is called the controllable canonical form uw linear systems (x. Learn how to obtain controllable.
Lecture 3 State Space Canonical forms YouTube
This realization is called the controllable canonical form uw linear systems (x. We will see that there are multiple models (or realizations) that correspond to the same transfer function. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. Theorem (kalman canonical form (controllability)) let x 2rn, x(k.
Controllable Canonical Phase Variable Form Method 1 Converting
This realization is called the controllable canonical form uw linear systems (x. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. We will see that there are multiple models (or realizations) that correspond to the same transfer function. Theorem (kalman canonical form (controllability)) let x 2rn, x(k.
State Space Introduction Controllable Canonical Form YouTube
We will see that there are multiple models (or realizations) that correspond to the same transfer function. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. This realization is called the controllable canonical form uw linear systems (x. Theorem (kalman canonical form (controllability)) let x 2rn, x(k.
LCS 53a Controllable Canonical Form (CCF) statespace models YouTube
Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. We will see that there are multiple models (or realizations) that correspond to the same transfer function. This realization is called the controllable canonical form uw linear systems (x. Theorem (kalman canonical form (controllability)) let x 2rn, x(k.
This Realization Is Called The Controllable Canonical Form Uw Linear Systems (X.
Theorem (kalman canonical form (controllability)) let x 2rn, x(k + 1) = ax(k) + bu(k), y(k) = cx(k) + du(k) be uncontrollable with rank of the. Learn how to obtain controllable canonical form, a minimal realization of a system with the characteristic polynomial in the a matrix, using. We will see that there are multiple models (or realizations) that correspond to the same transfer function.

.jpg)
.jpg)