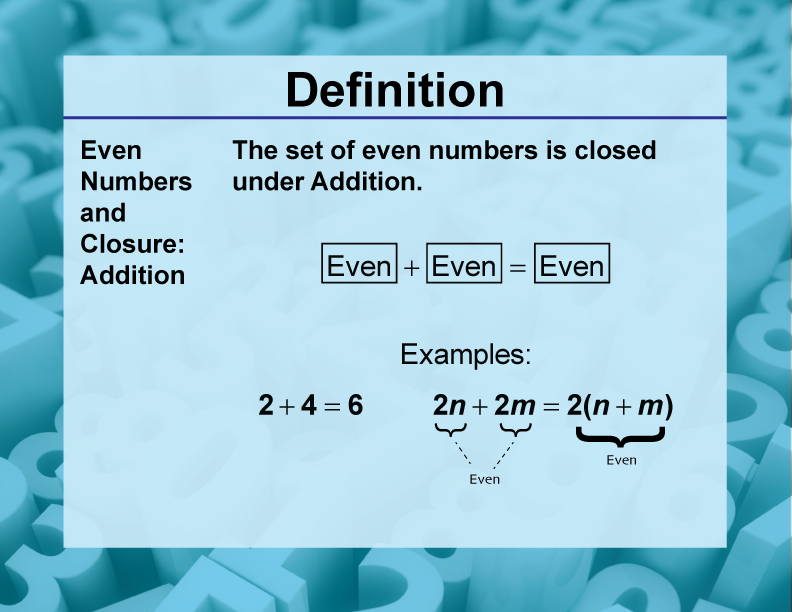
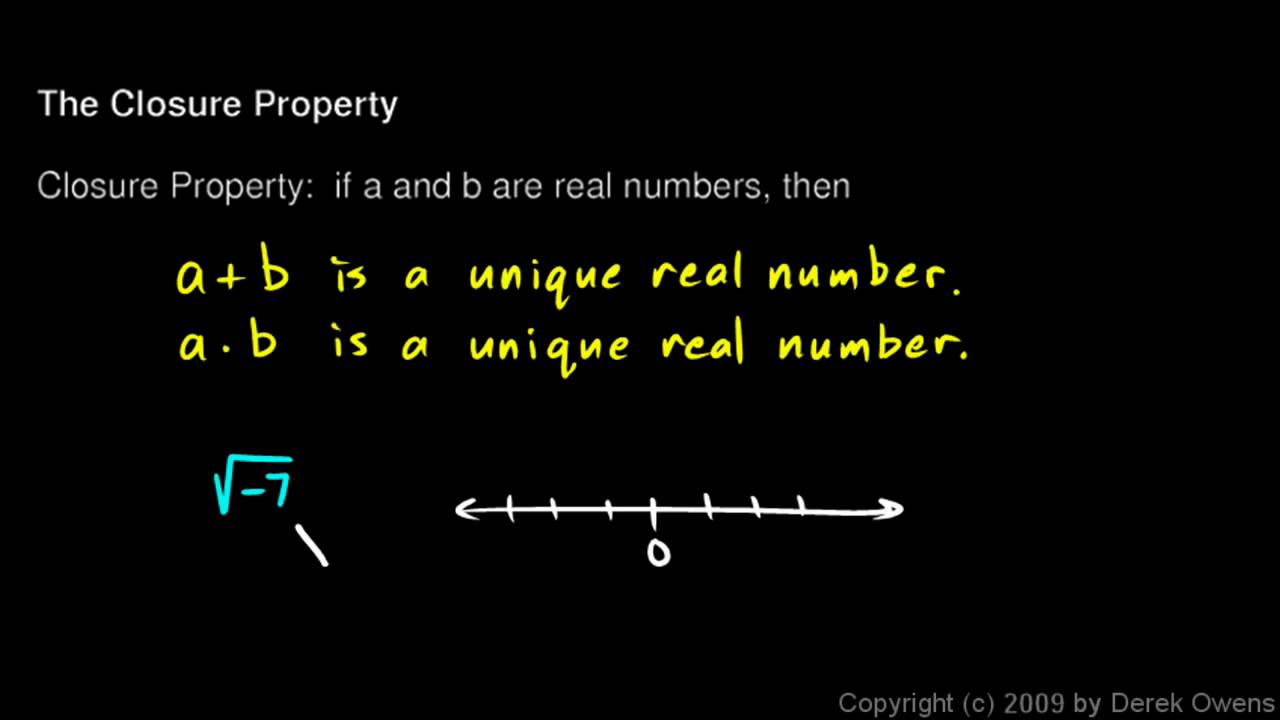
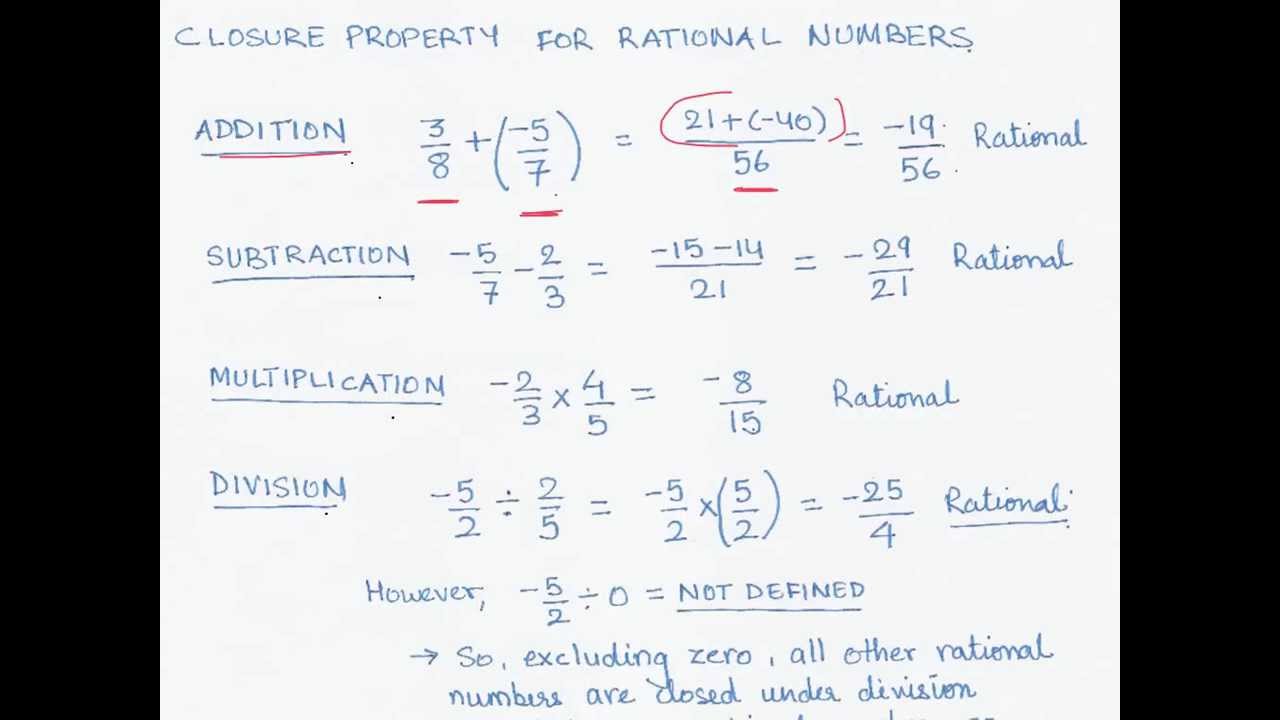
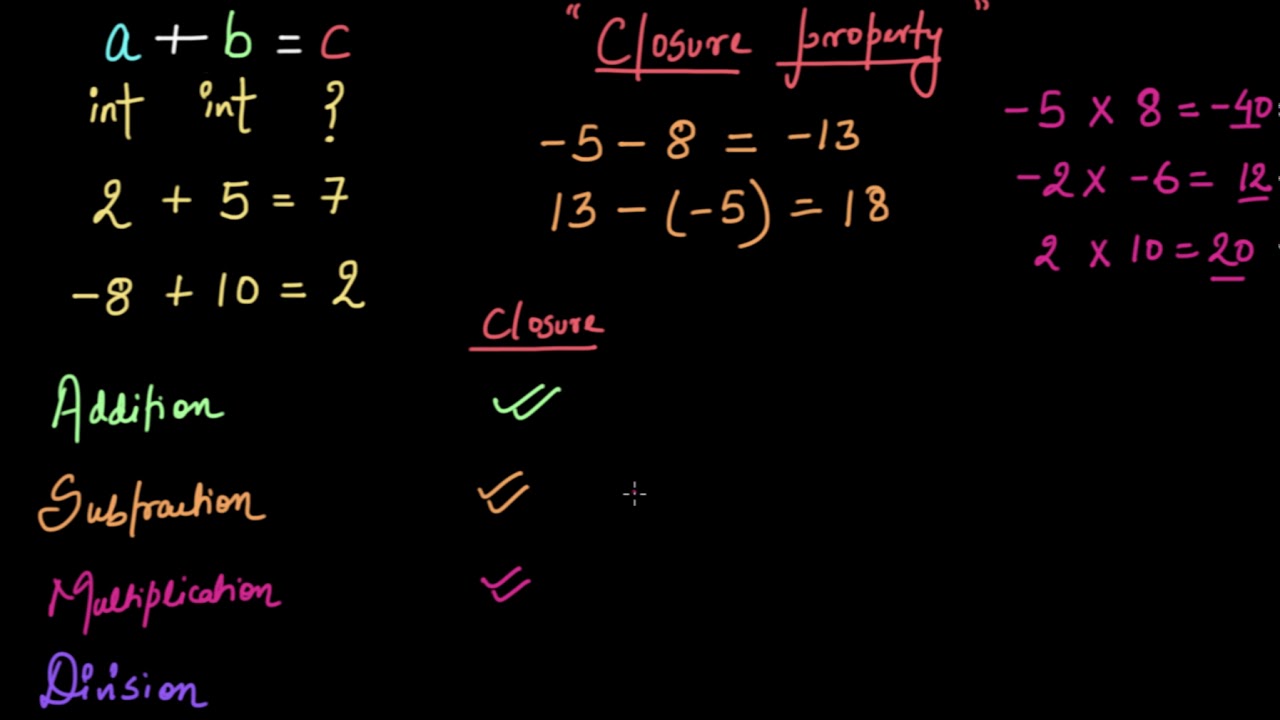Closure Math Property - Closure property holds for addition and multiplication of whole numbers. A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. Closure property of whole numbers under addition:
Closure property holds for addition and multiplication of whole numbers. Closure property of whole numbers under addition: Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set.
A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. Closure property of whole numbers under addition: Closure property holds for addition and multiplication of whole numbers.
DefinitionClosure Property TopicsEven Numbers and Closure Addition
Closure property of whole numbers under addition: Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. Closure property holds for addition and multiplication of whole numbers. A set is closed (under an operation) if and only if the operation on any two elements of.
Closure Property Of Addition Definition slidesharetrick
Closure property holds for addition and multiplication of whole numbers. Closure property of whole numbers under addition: A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure is when an operation (such as adding) on members of a set (such as real.
Properties of Integers Closure Property MathsMD
Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure property of whole numbers under addition: Closure.
Math Definitions Collection Closure Properties Media4Math
A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure property holds for addition and multiplication of whole numbers. Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the.
What Is Closure Property Definition, Formula, Examples
A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. Closure property holds for addition and multiplication of.
Integers closure property of multiplication YouTube
Closure property of whole numbers under addition: Closure property holds for addition and multiplication of whole numbers. A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure is when an operation (such as adding) on members of a set (such as real.
Algebra 1 2.01d The Closure Property YouTube
A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure property holds for addition and multiplication of whole numbers. Closure property of whole numbers under addition: Closure is when an operation (such as adding) on members of a set (such as real.
04 Proving Closure Property For Rational numbers YouTube
A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure property holds for addition and multiplication of whole numbers. Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the.
Whole Numbers(Part1) Closure Property of Whole Numbers MathsGrade7
Closure property of whole numbers under addition: A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure property holds for addition and multiplication of whole numbers. Closure is when an operation (such as adding) on members of a set (such as real.
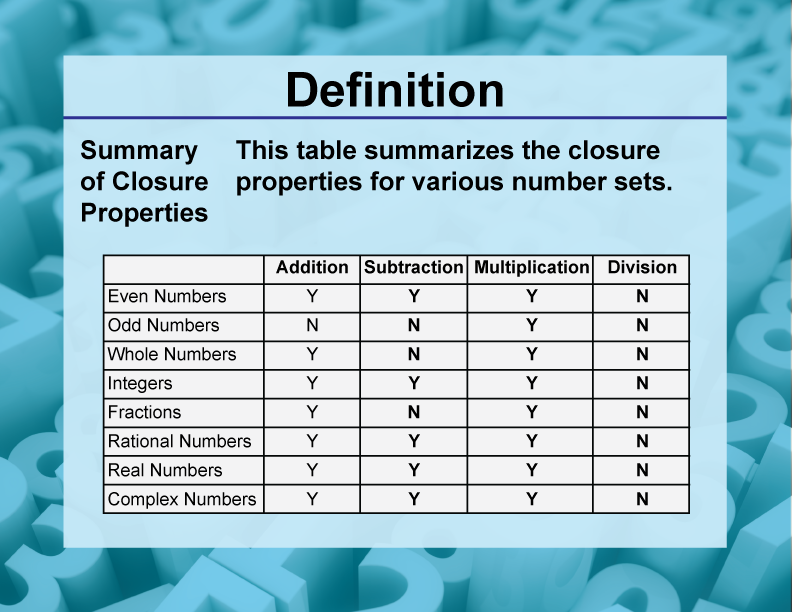
DefinitionClosure Property TopicsSummary of Closure Properties
Closure property holds for addition and multiplication of whole numbers. A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set. Closure property of whole numbers under addition: Closure is when an operation (such as adding) on members of a set (such as real.
Closure Property Of Whole Numbers Under Addition:
Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set. Closure property holds for addition and multiplication of whole numbers. A set is closed (under an operation) if and only if the operation on any two elements of the set produces another element of the same set.